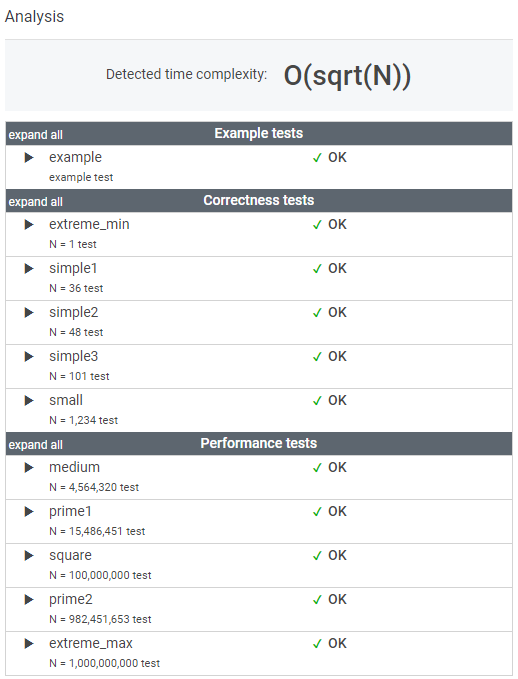
Problem
n integer N is given, representing the area of some rectangle.
The area of a rectangle whose sides are of length A and B is A * B, and the perimeter is 2 * (A + B).
The goal is to find the minimal perimeter of any rectangle whose area equals N. The sides of this rectangle should be only integers.
For example, given integer N = 30, rectangles of area 30 are:
- (1, 30), with a perimeter of 62,
- (2, 15), with a perimeter of 34,
- (3, 10), with a perimeter of 26,
- (5, 6), with a perimeter of 22.
Write a function:
int solution(int N);
that, given an integer N, returns the minimal perimeter of any rectangle whose area is exactly equal to N.
For example, given an integer N = 30, the function should return 22, as explained above.
Write an efficient algorithm for the following assumptions:
- N is an integer within the range [1..1,000,000,000].
Copyright 2009–2021 by Codility Limited. All Rights Reserved. Unauthorized copying, publication or disclosure prohibited.
How to solve
- 정보
정수N: 사각형의 넓이
가로 세로 길이 A, B사각형의 넓이를 나타내는 정수 N이 주어질 때,
넓이 Area N = A*B,
둘레 Perimeter = 2*(A+B) 로 표현할 수 있다.
- 구해야 하는것?
사각형의 넓이를 N으로하는 사각형중, 최소 perimeter(둘레)를 가지는 사각형의 perimeter를 return 한다.
- 예시
For example, given integer N = 30, rectangles of area 30 are:
- (1, 30), with a perimeter of 62,
- (2, 15), with a perimeter of 34,
- (3, 10), with a perimeter of 26,
- (5, 6), with a perimeter of 22.
문제에 나온 예를 보면 N=30일때,
A, B 길이가 아래와 같은 사각형을 만들 수 있고, 각각의 둘레의 길이는 아래와 같다.
(1, 30) 인 경우, 62
(2, 15) 인 경우, 34
(3, 10) 인 경우, 26
(5, 6) 인 경우, 22
즉, N=30일 때, 4개의 사각형이 만들어질 수 있는데,
최소 둘레를 가지는 사각형은 가로, 세로 길이 (5, 6)인 둘레 22의 사각형이다.
따라서 이 경우 22를 return 한다.
- 풀이
넓이가 N인 사각형의 변의 길이를 각각 가로 A, 세로 B라고 할때,
N = A*B로 표현할 수 있다.
1) 가능한 A 구하기
A<B라고 가정할 때,
가능한 A는 A<=sqrt(N) 이며, N%A == 0 인 정수이다.
2) A에 따른 B구하기
B = N/A
3) perimeter 구하기
perimeter = 2*(A+B)
4) 최소 perimeter 업데이트
perimeter_min = min(perimeter, perimeter_min)
Solution(c++)
// you can use includes, for example:
#include <bits/stdc++.h>
// you can write to stdout for debugging purposes, e.g.
// cout << "this is a debug message" << endl;
int solution(int N) {
// write your code in C++14 (g++ 6.2.0)
int perimeter_min = INT_MAX;
if(N == 1){
return 4;
}
for(int i=1; i<=sqrt(N); i++){
if(N%i == 0){
int A = i;
int B = N/i;
int perimeter = 2*(A+B);
perimeter_min = min(perimeter_min, perimeter);
}
}
return perimeter_min;
}Test Result
app.codility.com/demo/results/trainingTY3CVW-REV/
Test results - Codility
An integer N is given, representing the area of some rectangle. The area of a rectangle whose sides are of length A and B is A * B, and the perimeter is 2 * (A + B). The goal is to find the minimal perimeter of any rectangle whose area equals N. The sides
app.codility.com


'SW > 알고리즘 문제풀이' 카테고리의 다른 글
| [Codility] Lesson12 - Euclidean algorithm: Common Prime Divisors (0) | 2021.02.09 |
|---|---|
| [Codility] Lesson12 - euclidean algorithm: Chocolates By Numbers (1) | 2021.02.08 |
| [Atcoder] D - Friends (C++) (1) | 2021.01.13 |
| [Codility] Lesson10 - Prime and Composite numbers : CountFactors (c++) (1) | 2021.01.12 |
| [Codility] Lesson9 - Maximum Slice Problem: MaxSliceSum (c++) (0) | 2021.01.10 |


댓글